Answer:
Option A
Explanation:
Central Idea: The ray will get transmitted through face AC if $i_{AC}<i_{C}$ . Consider the ray diagram is shown below
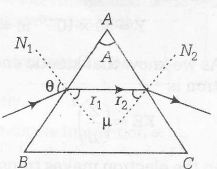
A ray of light incident on face AB at an angle θ
$r_{1}$ = Angle of refraction on face AB
$r_{2}$ = Angle of incidence at face AC
For transmission of light through face AC
$i_{AC}<i_{C}$ or $A-r_{1}<i_{C}$
or $\sin (A-r_{1})<\sin i_{C}$
or $\sin (A-r_{1})<\frac{1}{\mu}$
$A-r_{1}<\sin ^{-1}\left(\frac{1}{\mu}\right)$
or $\sin r_{1}>\sin \left[A-\sin ^{-1}\left(\frac{1}{\mu}\right)\right]$
Now, applying snell's law at the face AB
$1\times \sin \theta=\mu \sin r_{1}$ or $ \sin r_{1}=\frac{\sin \theta}{\mu}$
$\Rightarrow \frac{\sin \theta}{\mu}>\sin \left[A-\sin ^{-1}\left( \frac{1}{\mu}\right)\right]$
or $\theta >\sin ^{-1}\left[\mu \sin\left\{A-\sin ^{-1}\left(\frac{1}{\mu}\right)\right\}\right]$